Instruction
Write down the given logarithmic expression. If the expression uses the logarithm of 10, then its notation is shortened and looks like this: lg b is the decimal logarithm. If the logarithm has the number e as the base, then the expression is written: ln b is the natural logarithm. It is understood that the result of any is the power to which the base number must be raised to get the number b.
When finding two functions from the sum, you just need to differentiate them one by one, and add the results: (u+v)" = u"+v";
When finding the derivative of the product of two functions, it is necessary to multiply the derivative of the first function by the second and add the derivative of the second function, multiplied by the first function: (u*v)" = u"*v+v"*u;
In order to find the derivative of the quotient of two functions, it is necessary, from the product of the derivative of the dividend multiplied by the divisor function, to subtract the product of the derivative of the divisor multiplied by the divisor function, and divide all this by the divisor function squared. (u/v)" = (u"*v-v"*u)/v^2;
If a complex function is given, then it is necessary to multiply the derivative of the inner function and the derivative of the outer one. Let y=u(v(x)), then y"(x)=y"(u)*v"(x).
Using the obtained above, you can differentiate almost any function. So let's look at a few examples:
y=x^4, y"=4*x^(4-1)=4*x^3;
y=2*x^3*(e^x-x^2+6), y"=2*(3*x^2*(e^x-x^2+6)+x^3*(e^x-2 *x));
There are also tasks for calculating the derivative at a point. Let the function y=e^(x^2+6x+5) be given, you need to find the value of the function at the point x=1.
1) Find the derivative of the function: y"=e^(x^2-6x+5)*(2*x +6).
2) Calculate the value of the function at the given point y"(1)=8*e^0=8
Related videos
Helpful advice
Learn the table of elementary derivatives. This will save a lot of time.
Sources:
- constant derivative
So what is the difference between an irrational equation and a rational one? If the unknown variable is under the square root sign, then the equation is considered irrational.
Instruction
The main method for solving such equations is the method of raising both sides equations into a square. However. this is natural, the first step is to get rid of the sign. Technically, this method is not difficult, but sometimes it can lead to trouble. For example, the equation v(2x-5)=v(4x-7). By squaring both sides, you get 2x-5=4x-7. Such an equation is not difficult to solve; x=1. But the number 1 will not be given equations. Why? Substitute the unit in the equation instead of the x value. And the right and left sides will contain expressions that do not make sense, that is. Such a value is not valid for a square root. Therefore, 1 is an extraneous root, and therefore this equation has no roots.
So, the irrational equation is solved using the method of squaring both of its parts. And having solved the equation, it is necessary to cut off extraneous roots. To do this, substitute the found roots in the original equation.
Consider another one.
2x+vx-3=0
Of course, this equation can be solved using the same equation as the previous one. Transfer Compounds equations, which do not have a square root, to the right side and then use the squaring method. solve the resulting rational equation and roots. But another, more elegant one. Enter a new variable; vx=y. Accordingly, you will get an equation like 2y2+y-3=0. That is the usual quadratic equation. Find its roots; y1=1 and y2=-3/2. Next, solve two equations vx=1; vx \u003d -3/2. The second equation has no roots, from the first we find that x=1. Do not forget about the need to check the roots.
Solving identities is quite easy. This requires making identical transformations until the goal is achieved. Thus, with the help of the simplest arithmetic operations, the task will be solved.
You will need
- - paper;
- - pen.
Instruction
The simplest such transformations are algebraic abbreviated multiplications (such as the square of the sum (difference), the difference of squares, the sum (difference), the cube of the sum (difference)). In addition, there are many trigonometric formulas that are essentially the same identities.
Indeed, the square of the sum of two terms is equal to the square of the first plus twice the product of the first and the second plus the square of the second, that is, (a+b)^2= (a+b)(a+b)=a^2+ab +ba+b ^2=a^2+2ab+b^2.
Simplify Both
General principles of solution
Repeat from a textbook on mathematical analysis or higher mathematics, which is a definite integral. As you know, the solution of a definite integral is a function whose derivative will give an integrand. This function is called antiderivative. According to this principle, the basic integrals are constructed.Determine by the form of the integrand which of the table integrals is suitable in this case. It is not always possible to determine this immediately. Often, the tabular form becomes noticeable only after several transformations to simplify the integrand.
Variable substitution method
If the integrand is a trigonometric function whose argument is some polynomial, then try using the change of variables method. To do this, replace the polynomial in the argument of the integrand with some new variable. Based on the ratio between the new and old variable, determine the new limits of integration. By differentiating this expression, find a new differential in . Thus, you will get a new form of the old integral, close or even corresponding to any tabular one.Solution of integrals of the second kind
If the integral is an integral of the second kind, the vector form of the integrand, then you will need to use the rules for moving from these integrals to scalar ones. One such rule is the Ostrogradsky-Gauss ratio. This law makes it possible to pass from the rotor flow of some vector function to a triple integral over the divergence of a given vector field.Substitution of limits of integration
After finding the antiderivative, it is necessary to substitute the limits of integration. First, substitute the value of the upper limit into the expression for the antiderivative. You will receive some number. Next, subtract from the resulting number another number, the resulting lower limit to the antiderivative. If one of the integration limits is infinity, then when substituting it into the antiderivative function, it is necessary to go to the limit and find what the expression tends to.If the integral is two-dimensional or three-dimensional, then you will have to represent the geometric limits of integration in order to understand how to calculate the integral. Indeed, in the case of, say, a three-dimensional integral, the limits of integration can be entire planes that limit the volume to be integrated.
Definition of logarithm
The logarithm of the number b to the base a is the exponent to which you need to raise a to get b.
The number e in mathematics, it is customary to denote the limit to which the expression tends to
Number e is irrational number- a number incommensurable with one, it cannot be exactly expressed either as a whole or as a fraction rational number.
Letter e- the first letter of a Latin word exonere- to flaunt, hence the name in mathematics exponential- exponential function.
Number e widely used in mathematics, and in all sciences, one way or another using mathematical calculations for their needs.
Logarithms. Properties of logarithms
Definition: The base logarithm of a positive number b is the exponent c to which the number a must be raised to obtain the number b.
Basic logarithmic identity:
7) Formula for transition to a new base:
lna = log e a, e ≈ 2.718…
Tasks and tests on the topic “Logarithms. Properties of logarithms»
- Logarithms - Important topics for repeating the exam in mathematics
To successfully complete tasks on this topic, you must know the definition of the logarithm, the properties of logarithms, the basic logarithmic identity, the definitions of decimal and natural logarithms. The main types of tasks on this topic are tasks for calculating and converting logarithmic expressions. Let's consider their solution on the following examples.
Solution: Using the properties of logarithms, we get
Solution: using the properties of the degree, we get
1) (2 2) log 2 5 =(2 log 2 5) 2 =5 2 =25
Properties of logarithms, formulations and proofs.
Logarithms have a number of characteristic properties. In this article, we will analyze the main properties of logarithms. Here we give their formulations, write down the properties of logarithms in the form of formulas, show examples of their application, and also give proofs of the properties of logarithms.
Page navigation.
Basic properties of logarithms, formulas
For ease of remembering and using, we present basic properties of logarithms as a list of formulas. In the next section, we give their formulations, proofs, examples of use, and necessary explanations.
and the property of the logarithm of the product of n positive numbers: log a (x 1 x 2 ... x n) \u003d log a x 1 + log a x 2 + ... + log a x n, a>0, a≠1, x 1 >0, x 2 >0, …, xn >0 .
, where a>0 , a≠1 , x>0 , y>0 .
, where a>0 , a≠1 , n is a natural number greater than one, b>0 .
, a>0 , a≠1 , b>0 , b≠1 .
, a>0 , a≠1 , b>0 , p and q are real numbers, q≠0 , in particular, for b=a we have 
.Statements and proofs of properties
We pass to the formulation and proof of the recorded properties of logarithms. All properties of logarithms are proved on the basis of the definition of the logarithm and the basic logarithmic identity that follows from it, as well as the properties of the degree.
Let's start with properties of the logarithm of unity. Its formulation is as follows: the logarithm of unity is equal to zero, that is, log a 1=0 for any a>0 , a≠1 . The proof is straightforward: since a 0 =1 for any a that satisfies the above conditions a>0 and a≠1 , then the proven equality log a 1=0 immediately follows from the definition of the logarithm.
Let's give examples of application of the considered property: log 3 1=0 , lg1=0 and .
Let's move on to the next property: the logarithm of a number equal to the base is equal to one, that is, log a a=1 for a>0 , a≠1 . Indeed, since a 1 =a for any a , then by the definition of the logarithm log a a=1 .
Examples of using this property of logarithms are log 5 5=1 , log 5.6 5.6 and lne=1 .
The logarithm of the power of a number equal to the base of the logarithm is equal to the exponent. This property of the logarithm corresponds to a formula of the form log a a p =p, where a>0 , a≠1 and p is any real number. This property follows directly from the definition of the logarithm. Note that it allows you to immediately specify the value of the logarithm, if it is possible to represent the number under the sign of the logarithm as a degree of base, we will talk more about this in the article calculating logarithms.
For example, log 2 2 7 =7 , log10 -4 =-4 and 
.
Logarithm of the product of two positive numbers x and y is equal to the product of the logarithms of these numbers: log a (x y)=log a x+log a y, a>0 , a≠1 . Let us prove the property of the logarithm of the product. Due to the properties of the degree a log a x + log a y =a log a x a log a y , and since by the main logarithmic identity a log a x =x and a log a y =y , then a log a x a log a y =x y . Thus, a log a x+log a y =x y , whence the required equality follows by the definition of the logarithm.
Let's show examples of using the property of the logarithm of the product: log 5 (2 3)=log 5 2+log 5 3 and 
.
The product logarithm property can be generalized to the product of a finite number n of positive numbers x 1 , x 2 , …, x n as log a (x 1 x 2 ... x n)= log a x 1 +log a x 2 +...+log a x n. This equality can be easily proved by the method of mathematical induction.
For example, the natural logarithm of a product can be replaced by the sum of three natural logarithms of the numbers 4 , e , and .
Logarithm of the quotient of two positive numbers x and y is equal to the difference between the logarithms of these numbers. The property of the quotient logarithm corresponds to a formula of the form , where a>0 , a≠1 , x and y are some positive numbers. The validity of this formula is proved like the formula for the logarithm of the product: since 
, then by the definition of the logarithm .
Here is an example of using this property of the logarithm: 
.
Let's move on to property of the logarithm of degree. The logarithm of a degree is equal to the product of the exponent and the logarithm of the modulus of the base of this degree. We write this property of the logarithm of the degree in the form of a formula: log a b p =p log a |b|, where a>0 , a≠1 , b and p are numbers such that the degree of b p makes sense and b p >0 .
We first prove this property for positive b . The basic logarithmic identity allows us to represent the number b as a log a b , then b p =(a log a b) p , and the resulting expression, due to the power property, is equal to a p log a b . So we arrive at the equality b p =a p log a b , from which, by the definition of the logarithm, we conclude that log a b p =p log a b .
It remains to prove this property for negative b . Here we note that the expression log a b p for negative b makes sense only for even exponents p (since the value of the degree b p must be greater than zero, otherwise the logarithm will not make sense), and in this case b p =|b| p . Then b p =|b| p =(a log a |b|) p =a p log a |b| , whence log a b p =p log a |b| .
For example, 
and ln(-3) 4 =4 ln|-3|=4 ln3 .
It follows from the previous property property of the logarithm from the root: the logarithm of the root of the nth degree is equal to the product of the fraction 1/n and the logarithm of the root expression, that is, where a>0, a≠1, n is a natural number greater than one, b>0.
The proof is based on an equality (see the definition of exponent with a fractional exponent), which is valid for any positive b , and the property of the logarithm of the degree: 
.
Here is an example of using this property: 
.
Now let's prove conversion formula to the new base of the logarithm kind 
. To do this, it suffices to prove the validity of the equality log c b=log a b log c a . The basic logarithmic identity allows us to represent the number b as a log a b , then log c b=log c a log a b . It remains to use the property of the logarithm of the degree: log c a log a b = log a b log c a . Thus, the equality log c b=log a b log c a is proved, which means that the formula for the transition to a new base of the logarithm is also proved .
Let's show a couple of examples of applying this property of logarithms: and 
.
The formula for moving to a new base allows you to move on to working with logarithms that have a “convenient” base. For example, it can be used to switch to natural or decimal logarithms so that you can calculate the value of the logarithm from a table of logarithms. The formula for the transition to a new base of the logarithm also allows in some cases to find the value of a given logarithm, when the values of some logarithms with other bases are known.
A special case of the transition formula to a new base of the logarithm for c=b of the form is often used. This shows that log a b and log b a are mutually inverse numbers. Eg, 
.
The formula is also often used, which is convenient when finding logarithm values. To confirm our words, we will show how the value of the logarithm of the form is calculated using it. We have 
. To prove the formula, it suffices to use the transition formula to the new base of the logarithm a: 
.
It remains to prove the comparison properties of logarithms.
Let's use the opposite method. Suppose that for a 1 >1 , a 2 >1 and a 1 2 and for 0 1 log a 1 b≤log a 2 b is true. By the properties of logarithms, these inequalities can be rewritten as 
And 
respectively, and from them it follows that log b a 1 ≤log b a 2 and log b a 1 ≥log b a 2, respectively. Then, by the properties of powers with the same bases, the equalities b log b a 1 ≥b log b a 2 and b log b a 1 ≥b log b a 2 must be satisfied, that is, a 1 ≥a 2 . Thus, we have arrived at a contradiction to the condition a 1 2 . This completes the proof.
Basic properties of logarithms
- Materials for the lesson
- Download all formulas
- log a x n = n log a x ;
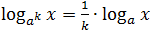
Logarithms, like any number, can be added, subtracted and converted in every possible way. But since logarithms are not quite ordinary numbers, there are rules here, which are called basic properties.
These rules must be known - no serious logarithmic problem can be solved without them. In addition, there are very few of them - everything can be learned in one day. So let's get started.
Addition and subtraction of logarithms
Consider two logarithms with the same base: log a x and log a y . Then they can be added and subtracted, and:
So, the sum of the logarithms is equal to the logarithm of the product, and the difference is the logarithm of the quotient. Please note: the key point here is - same grounds. If the bases are different, these rules do not work!
These formulas will help calculate the logarithmic expression even when its individual parts are not considered (see the lesson "What is a logarithm"). Take a look at the examples - and see:
Task. Find the value of the expression: log 6 4 + log 6 9.
Since the bases of logarithms are the same, we use the sum formula:
log 6 4 + log 6 9 = log 6 (4 9) = log 6 36 = 2.
Task. Find the value of the expression: log 2 48 − log 2 3.
The bases are the same, we use the difference formula:
log 2 48 - log 2 3 = log 2 (48: 3) = log 2 16 = 4.
Task. Find the value of the expression: log 3 135 − log 3 5.
Again, the bases are the same, so we have:
log 3 135 − log 3 5 = log 3 (135: 5) = log 3 27 = 3.
As you can see, the original expressions are made up of "bad" logarithms, which are not considered separately. But after transformations quite normal numbers turn out. Many tests are based on this fact. Yes, that control - similar expressions in all seriousness (sometimes - with virtually no changes) are offered at the exam.
Removing the exponent from the logarithm
Now let's complicate the task a little. What if there is a degree in the base or argument of the logarithm? Then the exponent of this degree can be taken out of the sign of the logarithm according to the following rules:
It is easy to see that the last rule follows their first two. But it's better to remember it anyway - in some cases it will significantly reduce the amount of calculations.
Of course, all these rules make sense if the ODZ logarithm is observed: a > 0, a ≠ 1, x > 0. And one more thing: learn to apply all formulas not only from left to right, but also vice versa, i.e. you can enter the numbers before the sign of the logarithm into the logarithm itself. This is what is most often required.
Task. Find the value of the expression: log 7 49 6 .
Let's get rid of the degree in the argument according to the first formula:
log 7 49 6 = 6 log 7 49 = 6 2 = 12
Task. Find the value of the expression:
[Figure caption]
Note that the denominator is a logarithm whose base and argument are exact powers: 16 = 2 4 ; 49 = 72. We have:
[Figure caption]
I think the last example needs clarification. Where have logarithms gone? Until the very last moment, we work only with the denominator. They presented the base and the argument of the logarithm standing there in the form of degrees and took out the indicators - they got a “three-story” fraction.
Now let's look at the main fraction. The numerator and denominator have the same number: log 2 7. Since log 2 7 ≠ 0, we can reduce the fraction - 2/4 will remain in the denominator. According to the rules of arithmetic, the four can be transferred to the numerator, which was done. The result is the answer: 2.
Transition to a new foundation
Speaking about the rules for adding and subtracting logarithms, I specifically emphasized that they only work with the same bases. What if the bases are different? What if they are not exact powers of the same number?
Formulas for transition to a new base come to the rescue. We formulate them in the form of a theorem:
Let the logarithm log a x be given. Then for any number c such that c > 0 and c ≠ 1, the equality is true:
[Figure caption]
In particular, if we put c = x , we get:
[Figure caption]
It follows from the second formula that it is possible to interchange the base and the argument of the logarithm, but in this case the whole expression is “turned over”, i.e. the logarithm is in the denominator.
These formulas are rarely found in ordinary numerical expressions. It is possible to evaluate how convenient they are only when solving logarithmic equations and inequalities.
However, there are tasks that cannot be solved at all except by moving to a new foundation. Let's consider a couple of these:
Task. Find the value of the expression: log 5 16 log 2 25.
Note that the arguments of both logarithms are exact exponents. Let's take out the indicators: log 5 16 = log 5 2 4 = 4log 5 2; log 2 25 = log 2 5 2 = 2log 2 5;
Now let's flip the second logarithm:
[Figure caption]
Since the product does not change from permutation of factors, we calmly multiplied four and two, and then figured out the logarithms.
Task. Find the value of the expression: log 9 100 lg 3.
The base and argument of the first logarithm are exact powers. Let's write it down and get rid of the indicators:
[Figure caption]
Now let's get rid of the decimal logarithm by moving to a new base:
[Figure caption]
Basic logarithmic identity
Often in the process of solving it is required to represent a number as a logarithm to a given base. In this case, the formulas will help us:
- n = log a a n
-
In the first case, the number n becomes the exponent in the argument. The number n can be absolutely anything, because it's just the value of the logarithm.
The second formula is actually a paraphrased definition. It's called the basic logarithmic identity.
Indeed, what will happen if the number b is raised to such a power that the number b to this power gives the number a? That's right: this is the same number a . Read this paragraph carefully again - many people "hang" on it.
Like the new base conversion formulas, the basic logarithmic identity is sometimes the only possible solution.
[Figure caption]
Note that log 25 64 = log 5 8 - just take the square of the base and the argument of the logarithm. Given the rules for multiplying powers with the same base, we get:
[Figure caption]
If someone is not in the know, this was a real task from the Unified State Examination 🙂
Logarithmic unit and logarithmic zero
In conclusion, I will give two identities that are difficult to call properties - rather, these are consequences from the definition of the logarithm. They are constantly found in problems and, surprisingly, create problems even for "advanced" students.
- log a a = 1 is the logarithmic unit. Remember once and for all: the logarithm to any base a from this base itself is equal to one.
- log a 1 = 0 is logarithmic zero. The base a can be anything, but if the argument is one - the logarithm is zero! Because a 0 = 1 is a direct consequence of the definition.
That's all the properties. Be sure to practice putting them into practice! Download the cheat sheet at the beginning of the lesson, print it out - and solve the problems.
Logarithm. Properties of the logarithm (addition and subtraction).
Properties of the logarithm follow from its definition. And so the logarithm of the number b by reason A defined as the exponent to which a number must be raised a to get the number b(the logarithm exists only for positive numbers).
From this formulation it follows that the calculation x=log a b, is equivalent to solving the equation ax=b. For example, log 2 8 = 3 because 8 = 2 3 . The formulation of the logarithm makes it possible to justify that if b=a c, then the logarithm of the number b by reason a equals With. It is also clear that the topic of logarithm is closely related to the topic of the power of a number.
With logarithms, as with any numbers, you can perform addition, subtraction operations and transform in every possible way. But in view of the fact that logarithms are not quite ordinary numbers, their own special rules apply here, which are called basic properties.
Addition and subtraction of logarithms.
Take two logarithms with the same base: log x And log a y. Then remove it is possible to perform addition and subtraction operations:
As we see, sum of logarithms equals the logarithm of the product, and difference logarithms- the logarithm of the quotient. And this is true if the numbers A, X And at positive and a ≠ 1.
It is important to note that the main aspect in these formulas are the same bases. If the bases differ from each other, these rules do not apply!
The rules for adding and subtracting logarithms with the same bases are read not only from left to right, but also vice versa. As a result, we have the theorems for the logarithm of the product and the logarithm of the quotient.
Logarithm of the product two positive numbers is equal to the sum of their logarithms ; paraphrasing this theorem, we get the following, if the numbers A, x And at positive and a ≠ 1, That:
Logarithm of the quotient of two positive numbers is equal to the difference between the logarithms of the dividend and the divisor. In other words, if the numbers A, X And at positive and a ≠ 1, That:
We apply the above theorems to solve examples:
If numbers x And at are negative, then product logarithm formula becomes meaningless. So, it is forbidden to write:
since the expressions log 2 (-8) and log 2 (-4) are not defined at all (the logarithmic function at= log 2 X defined only for positive values of the argument X).
Product theorem is applicable not only for two, but also for an unlimited number of factors. This means that for every natural k and any positive numbers x 1 , x 2 , . . . ,x n there is an identity:
From quotient logarithm theorems one more property of the logarithm can be obtained. It is well known that log a 1= 0, therefore,
So there is an equality:
Logarithms of two mutually reciprocal numbers on the same basis will differ from each other only in sign. So:
Logarithm. Properties of logarithms
Logarithm. Properties of logarithms
Consider equality. Let us know the values and and we want to find the value of .
That is, we are looking for an exponent to which you need to cock to get .
Let
the variable can take any real value, then the following restrictions are imposed on the variables: o” title=”a>o”/> , 1″ title=”a1″/>, 0″ title=”b>0″/>If we know the values \u200b\u200bof and , and we are faced with the task of finding the unknown, then for this purpose a mathematical operation is introduced, which is called logarithm.
To find the value we take logarithm of a number By foundation :
The logarithm of a number to the base is the exponent to which you need to raise to get .
That is basic logarithmic identity:
o” title=”a>o”/> , 1″ title=”a1″/>, 0″ title=”b>0″/>
is essentially mathematical notation logarithm definitions.
The mathematical operation logarithm is the inverse of exponentiation, so properties of logarithms are closely related to the properties of the degree.
We list the main properties of logarithms:
(o” title=”a>o”/> , 1″ title=”a1″/>, 0″ title=”b>0″/>, 0,
d>0″/>, 1″ title=”d1″/>
4.
5.
The following group of properties allows you to represent the exponent of the expression under the sign of the logarithm, or standing at the base of the logarithm as a coefficient before the sign of the logarithm:
6.
7.
8.
9.
The next group of formulas allows you to go from a logarithm with a given base to a logarithm with an arbitrary base, and is called transition formulas to a new base:
10.
12. (corollary from property 11)
The following three properties are not well known, but they are often used when solving logarithmic equations, or when simplifying expressions containing logarithms:
13.
14.
15.
Special cases:
— decimal logarithm
— natural logarithmWhen simplifying expressions containing logarithms, a general approach is applied:
1. We represent decimal fractions in the form of ordinary ones.
2. We represent mixed numbers as improper fractions.
3. The numbers at the base of the logarithm and under the sign of the logarithm are decomposed into prime factors.
4. We try to bring all logarithms to the same base.
5. Apply the properties of logarithms.
Let's look at examples of simplifying expressions containing logarithms.
Example 1
Calculate:
Let's simplify all the exponents: our task is to bring them to logarithms, the base of which is the same number as the base of the exponent.
==(by property 7)=(by property 6) =
Substitute the indicators that we have obtained in the original expression. We get:
Answer: 5.25
Example 2 Calculate:
We bring all logarithms to base 6 (in this case, the logarithms from the denominator of the fraction will “migrate” to the numerator):
Let's decompose the numbers under the sign of the logarithm into prime factors:
Apply properties 4 and 6:
We introduce the replacement
We get:
Answer: 1
Logarithm . Basic logarithmic identity.
Properties of logarithms. Decimal logarithm. natural logarithm.
logarithm positive number N in base (b > 0, b 1) is called the exponent x to which you need to raise b to get N .
This entry is equivalent to the following: b x = N .
EXAMPLES: log 3 81 = 4 since 3 4 = 81 ;
log 1/3 27 = – 3 because (1/3) - 3 = 3 3 = 27 .
The above definition of the logarithm can be written as an identity:
Basic properties of logarithms.
2) log 1 = 0 because b 0 = 1 .
3) The logarithm of the product is equal to the sum of the logarithms of the factors:
4) The logarithm of the quotient is equal to the difference between the logarithms of the dividend and the divisor:
5) The logarithm of the degree is equal to the product of the exponent and the logarithm of its base:
The consequence of this property is the following: log root equals the logarithm of the root number divided by the power of the root:
6) If the base of the logarithm is a degree, then the value the reciprocal of the exponent can be taken out of the rhyme log sign:
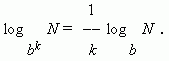
The last two properties can be combined into one:
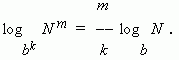
7) The formula for the transition modulus (i.e. the transition from one base of the logarithm to another base):
In a particular case, when N = a we have:
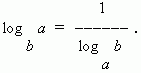
Decimal logarithm called base logarithm 10. It is denoted lg, i.e. log 10 N= log N. Logarithms of numbers 10, 100, 1000, . p are 1, 2, 3, …, respectively, i.e. have so many positive
units, how many zeros are in the logarithm number after one. Logarithms of numbers 0.1, 0.01, 0.001, . p are –1, –2, –3, …, respectively, i.e. have as many negative ones as there are zeros in the logarithm number before the one (including zero integers). The logarithms of the remaining numbers have a fractional part called mantissa. The integer part of the logarithm is called characteristic. For practical applications, decimal logarithms are most convenient.
natural logarithm called base logarithm e. It is denoted by ln, i.e. log e N=ln N. Number e is irrational, its approximate value is 2.718281828. It is the limit towards which the number (1 + 1 / n) n with unlimited increase n(cm. first wonderful limit on the Number Sequence Limits page).
Strange as it may seem, natural logarithms turned out to be very convenient when carrying out various operations related to the analysis of functions. Calculating base logarithms e much faster than any other basis.
- What do you need today to adopt a child in Russia? Adoption in Russia, in addition to a responsible personal decision, involves a number of procedures for state verification of candidates. Rigid selection at the preparatory stage contributes to more […]
- Information free of charge by TIN or OGRN from the tax register throughout Russia - online On the Unified Portal of Tax Services, information on state registration of legal entities, individual entrepreneurs, […]
- Punishment for driving without documents (driver's license, insurance, STS) Sometimes, due to forgetfulness, drivers get behind the wheel without a license and receive a fine for driving without documents. Recall that a motorist driving with him without fail […]
- Flowers for men. What kind of flowers can you give a man? What flowers can be given to a man? There are not so many "male" flowers, but there are those that are given to men. A small list of flowers in front of you: Chrysanthemums. Roses. Carnations. […]
- A memo is a special form of a document that is used in the internal environment of an enterprise and serves to quickly solve current production problems. Usually this document is drawn up for the purpose of making some […]
- When and how to get the funded part of the pension in Sberbank? Sberbank is a partner bank of the state pension fund. On the basis of this, citizens who have issued a funded pension could transfer the funded […]
- Child allowances in Ulyanovsk and the Ulyanovsk region in 2018 In addition, programs approved by federal law are operating in all regions. Let's see who and what benefits can count on. As regional authorities […]
- Detailed guide on how to draw up a power of attorney to represent the interests of an individual in court In a civil or arbitration lawsuit, in an administrative or criminal case, the interests of both the plaintiff and the defendant can be represented by an attorney: […]
As you know, when multiplying expressions with powers, their exponents always add up (a b * a c = a b + c). This mathematical law was derived by Archimedes, and later, in the 8th century, the mathematician Virasen created a table of integer indicators. It was they who served for the further discovery of logarithms. Examples of using this function can be found almost everywhere where it is required to simplify cumbersome multiplication to simple addition. If you spend 10 minutes reading this article, we will explain to you what logarithms are and how to work with them. Simple and accessible language.
Definition in mathematics
The logarithm is an expression of the following form: log a b=c, that is, the logarithm of any non-negative number (that is, any positive) "b" according to its base "a" is considered the power of "c", to which it is necessary to raise the base "a", so that in the end get the value "b". Let's analyze the logarithm using examples, let's say there is an expression log 2 8. How to find the answer? It's very simple, you need to find such a degree that from 2 to the required degree you get 8. Having done some calculations in your mind, we get the number 3! And rightly so, because 2 to the power of 3 gives the number 8 in the answer.
Varieties of logarithms
For many pupils and students, this topic seems complicated and incomprehensible, but in fact, logarithms are not so scary, the main thing is to understand their general meaning and remember their properties and some rules. There are three distinct kinds of logarithmic expressions:
- Natural logarithm ln a, where the base is the Euler number (e = 2.7).
- Decimal a, where the base is 10.
- The logarithm of any number b to the base a>1.
Each of them is solved in a standard way, including simplification, reduction and subsequent reduction to one logarithm using logarithmic theorems. To obtain the correct values of logarithms, one should remember their properties and the order of actions in their decisions.
Rules and some restrictions
In mathematics, there are several rules-limitations that are accepted as an axiom, that is, they are not subject to discussion and are true. For example, it is impossible to divide numbers by zero, and it is also impossible to extract the root of an even degree from negative numbers. Logarithms also have their own rules, following which you can easily learn how to work even with long and capacious logarithmic expressions:
- the base "a" must always be greater than zero, and at the same time not be equal to 1, otherwise the expression will lose its meaning, because "1" and "0" to any degree are always equal to their values;
- if a > 0, then a b > 0, it turns out that "c" must be greater than zero.
How to solve logarithms?
For example, the task was given to find the answer to the equation 10 x \u003d 100. It is very easy, you need to choose such a power, raising the number ten to which we get 100. This, of course, is 10 2 \u003d 100.
Now let's represent this expression as a logarithmic one. We get log 10 100 = 2. When solving logarithms, all actions practically converge to finding the degree to which the base of the logarithm must be entered in order to obtain a given number.
To accurately determine the value of an unknown degree, you must learn how to work with a table of degrees. It looks like this:
As you can see, some exponents can be guessed intuitively if you have a technical mindset and knowledge of the multiplication table. However, larger values will require a power table. It can be used even by those who do not understand anything at all in complex mathematical topics. The left column contains numbers (base a), the top row of numbers is the value of the power c, to which the number a is raised. At the intersection in the cells, the values of the numbers are determined, which are the answer (a c =b). Let's take, for example, the very first cell with the number 10 and square it, we get the value 100, which is indicated at the intersection of our two cells. Everything is so simple and easy that even the most real humanist will understand!
Equations and inequalities
It turns out that under certain conditions, the exponent is the logarithm. Therefore, any mathematical numerical expressions can be written as a logarithmic equation. For example, 3 4 =81 can be written as the logarithm of 81 to base 3, which is four (log 3 81 = 4). For negative powers, the rules are the same: 2 -5 = 1/32 we write as a logarithm, we get log 2 (1/32) = -5. One of the most fascinating sections of mathematics is the topic of "logarithms". We will consider examples and solutions of equations a little lower, immediately after studying their properties. Now let's look at what inequalities look like and how to distinguish them from equations.
An expression of the following form is given: log 2 (x-1) > 3 - it is a logarithmic inequality, since the unknown value "x" is under the sign of the logarithm. And also in the expression two quantities are compared: the logarithm of the desired number in base two is greater than the number three.
The most important difference between logarithmic equations and inequalities is that equations with logarithms (for example, the logarithm of 2 x = √9) imply one or more specific numerical values in the answer, while when solving the inequality, both the range of acceptable values and the points breaking this function. As a consequence, the answer is not a simple set of individual numbers, as in the answer of the equation, but a continuous series or set of numbers.
Basic theorems about logarithms
When solving primitive tasks on finding the values of the logarithm, its properties may not be known. However, when it comes to logarithmic equations or inequalities, first of all, it is necessary to clearly understand and apply in practice all the basic properties of logarithms. We will get acquainted with examples of equations later, let's first analyze each property in more detail.
- The basic identity looks like this: a logaB =B. It only applies if a is greater than 0, not equal to one, and B is greater than zero.
- The logarithm of the product can be represented in the following formula: log d (s 1 * s 2) = log d s 1 + log d s 2. In this case, the prerequisite is: d, s 1 and s 2 > 0; a≠1. You can give a proof for this formula of logarithms, with examples and a solution. Let log a s 1 = f 1 and log a s 2 = f 2, then a f1 = s 1 , a f2 = s 2. We get that s 1 *s 2 = a f1 *a f2 = a f1+f2 (properties of degrees ), and further by definition: log a (s 1 *s 2)= f 1 + f 2 = log a s1 + log a s 2, which was to be proved.
- The logarithm of the quotient looks like this: log a (s 1 / s 2) = log a s 1 - log a s 2.
- The theorem in the form of a formula takes the following form: log a q b n = n/q log a b.
This formula is called "property of the degree of the logarithm". It resembles the properties of ordinary degrees, and it is not surprising, because all mathematics rests on regular postulates. Let's look at the proof.
Let log a b \u003d t, it turns out a t \u003d b. If you raise both parts to the power m: a tn = b n ;
but since a tn = (a q) nt/q = b n , hence log a q b n = (n*t)/t, then log a q b n = n/q log a b. The theorem has been proven.
Examples of problems and inequalities
The most common types of logarithm problems are examples of equations and inequalities. They are found in almost all problem books, and are also included in the mandatory part of exams in mathematics. To enter a university or pass entrance tests in mathematics, you need to know how to solve such tasks correctly.
Unfortunately, there is no single plan or scheme for solving and determining the unknown value of the logarithm, however, certain rules can be applied to each mathematical inequality or logarithmic equation. First of all, you should find out whether the expression can be simplified or reduced to a general form. You can simplify long logarithmic expressions if you use their properties correctly. Let's get to know them soon.
When solving logarithmic equations, it is necessary to determine what kind of logarithm we have before us: an example of an expression may contain a natural logarithm or a decimal one.
Here are examples ln100, ln1026. Their solution boils down to the fact that you need to determine the degree to which the base 10 will be equal to 100 and 1026, respectively. For solutions of natural logarithms, one must apply logarithmic identities or their properties. Let's look at examples of solving logarithmic problems of various types.
How to Use Logarithm Formulas: With Examples and Solutions
So, let's look at examples of using the main theorems on logarithms.
- The property of the logarithm of the product can be used in tasks where it is necessary to decompose a large value of the number b into simpler factors. For example, log 2 4 + log 2 128 = log 2 (4*128) = log 2 512. The answer is 9.
- log 4 8 = log 2 2 2 3 = 3/2 log 2 2 = 1.5 - as you can see, using the fourth property of the degree of the logarithm, we managed to solve at first glance a complex and unsolvable expression. It is only necessary to factorize the base and then take the exponent values out of the sign of the logarithm.
Tasks from the exam
Logarithms are often found in entrance exams, especially a lot of logarithmic problems in the Unified State Exam (state exam for all school graduates). Usually these tasks are present not only in part A (the easiest test part of the exam), but also in part C (the most difficult and voluminous tasks). The exam implies an accurate and perfect knowledge of the topic "Natural logarithms".
Examples and problem solving are taken from the official versions of the exam. Let's see how such tasks are solved.
Given log 2 (2x-1) = 4. Solution:
let's rewrite the expression, simplifying it a bit log 2 (2x-1) = 2 2 , by the definition of the logarithm we get that 2x-1 = 2 4 , therefore 2x = 17; x = 8.5.
- All logarithms are best reduced to the same base so that the solution is not cumbersome and confusing.
- All expressions under the sign of the logarithm are indicated as positive, therefore, when taking out the exponent of the exponent of the expression, which is under the sign of the logarithm and as its base, the expression remaining under the logarithm must be positive.
derived from its definition. And so the logarithm of the number b by reason A defined as the exponent to which a number must be raised a to get the number b(the logarithm exists only for positive numbers).
From this formulation it follows that the calculation x=log a b, is equivalent to solving the equation ax=b. For example, log 2 8 = 3 because 8 = 2 3 . The formulation of the logarithm makes it possible to justify that if b=a c, then the logarithm of the number b by reason a equals With. It is also clear that the topic of the logarithm is closely related to the topic of the power of a number.
With logarithms, as with any numbers, you can perform operations of addition, subtraction and transform in every possible way. But in view of the fact that logarithms are not quite ordinary numbers, their own special rules apply here, which are called basic properties.
Addition and subtraction of logarithms.
Take two logarithms with the same base: log x And log a y. Then remove it is possible to perform addition and subtraction operations:
log a x+ log a y= log a (x y);
log a x - log a y = log a (x:y).
log a(x 1 . x 2 . x 3 ... x k) = log x 1 + log x 2 + log x 3 + ... + log a x k.
From quotient logarithm theorems one more property of the logarithm can be obtained. It is well known that log a 1= 0, therefore,
log a 1 /b= log a 1 - log a b= -log a b.
So there is an equality:
log a 1 / b = - log a b.
Logarithms of two mutually reciprocal numbers on the same basis will differ from each other only in sign. So:
Log 3 9= - log 3 1 / 9 ; log 5 1 / 125 = -log 5 125.
(from the Greek λόγος - "word", "relation" and ἀριθμός - "number") numbers b by reason a(log α b) is called such a number c, And b= a c, that is, log α b=c And b=ac are equivalent. The logarithm makes sense if a > 0, a ≠ 1, b > 0.
In other words logarithm numbers b by reason A formulated as an exponent to which a number must be raised a to get the number b(the logarithm exists only for positive numbers).
From this formulation it follows that the calculation x= log α b, is equivalent to solving the equation a x =b.
For example:
log 2 8 = 3 because 8=2 3 .
We note that the indicated formulation of the logarithm makes it possible to immediately determine logarithm value when the number under the sign of the logarithm is a certain power of the base. Indeed, the formulation of the logarithm makes it possible to justify that if b=a c, then the logarithm of the number b by reason a equals With. It is also clear that the topic of logarithm is closely related to the topic degree of number.
The calculation of the logarithm is referred to logarithm. Logarithm is the mathematical operation of taking a logarithm. When taking a logarithm, the products of factors are transformed into sums of terms.
Potentiation is the mathematical operation inverse to logarithm. When potentiating, the given base is raised to the power of the expression on which the potentiation is performed. In this case, the sums of terms are transformed into the product of factors.
Quite often, real logarithms with bases 2 (binary), e Euler number e ≈ 2.718 (natural logarithm) and 10 (decimal) are used.
At this stage, it is worth considering samples of logarithms log 7 2 , ln √ 5, lg0.0001.
And the entries lg (-3), log -3 3.2, log -1 -4.3 do not make sense, since in the first of them a negative number is placed under the sign of the logarithm, in the second - a negative number in the base, and in the third - and a negative number under the sign of the logarithm and unit in the base.
Conditions for determining the logarithm.
It is worth considering separately the conditions a > 0, a ≠ 1, b > 0. definition of a logarithm. Let's consider why these restrictions are taken. This will help us with an equality of the form x = log α b, called the basic logarithmic identity, which directly follows from the definition of the logarithm given above.
Take the condition a≠1. Since one is equal to one to any power, then the equality x=log α b can only exist when b=1, but log 1 1 will be any real number. To eliminate this ambiguity, we take a≠1.
Let us prove the necessity of the condition a>0. At a=0 according to the formulation of the logarithm, can only exist when b=0. And then accordingly log 0 0 can be any non-zero real number, since zero to any non-zero power is zero. To eliminate this ambiguity, the condition a≠0. And when a<0 we would have to reject the analysis of the rational and irrational values of the logarithm, since the exponent with a rational and irrational exponent is defined only for non-negative bases. It is for this reason that the condition a>0.
And the last condition b>0 follows from the inequality a>0, because x=log α b, and the value of the degree with a positive base a always positive.
Features of logarithms.
Logarithms characterized by distinctive features, which led to their widespread use to greatly facilitate painstaking calculations. In the transition "to the world of logarithms", multiplication is transformed into a much easier addition, division into subtraction, and raising to a power and taking a root are transformed into multiplication and division by an exponent, respectively.
The formulation of logarithms and a table of their values (for trigonometric functions) was first published in 1614 by the Scottish mathematician John Napier. Logarithmic tables, enlarged and detailed by other scientists, were widely used in scientific and engineering calculations, and remained relevant until electronic calculators and computers began to be used.
